- The design of lifting beams must meet specific calculation requirements per BTH-1 to verify that the design will be safe and effective for its intended below-the-hook lifting application. By using BTH-1 for designing lifting beams, the different failure modes of the beam will be addressed, and appropriate safety factors will be applied to the.
- The ClearCalcs beam calculator allows the user to input the geometry and loading of a beam for analysis in a few simple steps. It then determines bending moment, shear and deflection diagrams, and maximum demands using a powerful finite element analysis engine. Signing up for a ClearCalcs account will unlock further advanced features for design.
How to Use The Free Beam Calculator
The ClearCalcs beam calculator allows the user to input the geometry and loading of a beam for analysis in a few simple steps. It then determines bending moment, shear and deflection diagrams, and maximum demands using a powerful finite element analysis engine.
The term lifting beam is used for a beam suspended from a more central point so that the beam is loaded in bending, see figure 2. However many designs are a hybrid of the two. For the purposes of this guide, spreader beams, lifting beams and their hybrids are referred to under the generic name of lifting beams. Spreader Bar Lifting Device Calculations And Design. Designing A Spreader Beam For Lifting Thenavalarch. Sling Load And Beam Support Cable Tension Calculations Part 2. Capacity Of A Spreader Beam Ox Worldwide Heavy Lifting Equipment. Spreader Bar Design Process A To Designing Lifting And Beams Sparta Ering. To Lifting Beams And Spreaders.
Signing up for a ClearCalcs account will unlock further advanced features for design and analysis of beams and a variety of other structural elements. ClearCalcs enables design in steel, concrete and timber, according to Australian, US and EU Standards.
The sheet is divided into three main sections:
- ‘Key Properties’, where the user inputs the geometry of their chosen section and the beam supports.
- ‘Loads’, where the use can input distributed, point and applied moment loads,
- ‘Summary’, which displays the key outputs and diagrams.
A ‘Comments’ section is also included for the user to leave any specific design notes. Clicking on any of the input/property labels gives a descriptive reference explanation.
1. Input Key Properties
The properties of the beam and section are specified by typing directly into the input fields.
Length of Beam is the total including all spans of the beam, in mm or ft.
Young’s Modulus is set to a default value of 200,000 MPa or 29000 ksi for structural steel, but can be edited by the user.
Area of the Cross-Section is specific to the beam section selected, and is defaulted to the values for a common steel beam.

Second Moment of Area (or Moment of Inertia) is also specific to the beam section selected, and again defaulted to the properties of a common steel beam.
The properties E, A, and Ix for other beam sections can be obtained from the ClearCalcs section properties library. Alternately, you can create your own custom section using our free moment of inertia calculator.
Position of Supports from Left allow the user to input any number of supports, and specify their position along the length of the beam. The support type can either be pinned (fixed in translation, free in rotation) or fixed (fixed in both translation and rotation) and is selected from the drop-down menu. A minimum of one fixed support, or two pinned supports are required.
The beam calculator also allows cantilever spans at each end, as the position of the first support does not have to be equal to 0mm and the last support position does not have to be equal to the length of the beam.
The reactions at each of the supports are automatically updated as supports are added, changed or deleted, based on the specified loading.
2. Input Loads
The calculator supports a variety of different loading types which can be applied in combination. Each load can be named by the user.
The sign convention used for loading is (positive values shown):
Distributed Loads are specified in units of force per unit length, kN/m or plf, along the beam, and can be applied between any two points. Two different types can be applied in the calculator:
Uniform Loads have a constant magnitude along the length of application. Therefore, the start and end magnitudes specified by the user must be the same.
Linear Loads have a varying magnitude along the length of application. The different start and end magnitudes must be specified by the user, and they can be used to represent triangular or trapezoidal loads.
Point Loads are specified in units of force, kN or kip, and area applied at discrete points along the beam. For example, these can represent reactions from other members connecting to the beam. The user inputs the name, magnitude and location from the left of the beam.
The example diagram below, from the summary section, shows a two-span continuous beam with a linear distributed patch load and point load.
3. Calculation Summary Outputs
Once the loading and geometry have been specified, the calculator automatically uses the ClearCalcs finite element analysis engine to determine the moments, shear forces and deflections. The maximum values of each are output as ‘Moment Demand’, ’Shear Demand’ and ‘Deflection’, along with the diagrams along the length of the beam.
Positive values imply a downward deflection and negative values imply an upward deflection. The sign convention used in the shear force and bending moment diagrams is (positive values shown):
Using the cursor to hover over any point on the bending moment, shear force or deflection diagrams gives the specific values at that location along the beam. The example below shows the outputs for a two-span continuous beam with a linear distributed patch load and point load.
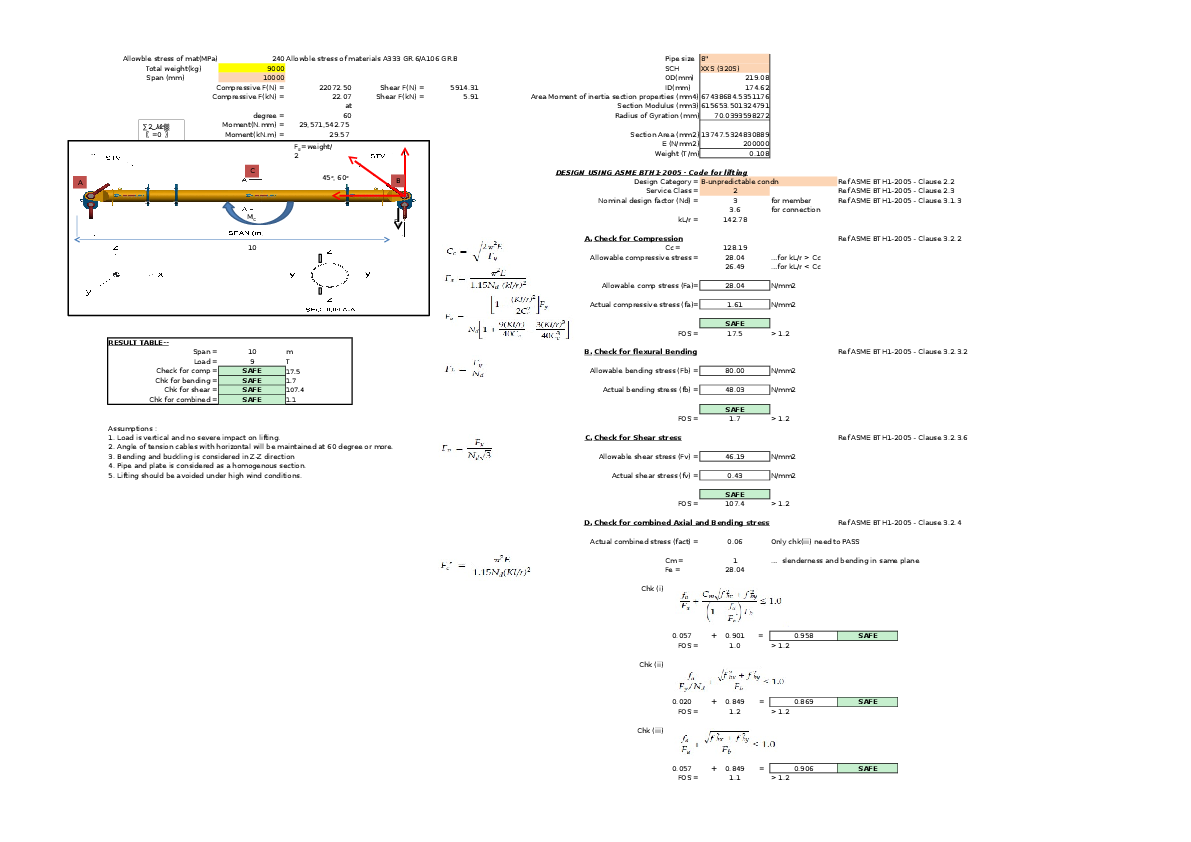
Below-the-hook lifting devices such as spreader bars and lifting beams must be designed in accordance with ASME BTH-1 and are to be manufactured and used in accordance with requirements of ASME B30.20 which specifies requirements for marking, inspection, construction and operation. The design of lifting beams must meet specific calculation requirements per BTH-1 to verify that the design will be safe and effective for its intended below-the-hook lifting application. By using BTH-1 for designing lifting beams, the different failure modes of the beam will be addressed, and appropriate safety factors will be applied to the design, dependent on the intended use or classification of the beam.
Lifting Device Configuration
Spreader Bar Inspection Criteria
The first step in developing a lifting device is to determine the lifting configuration, max lift capacity and max spread (width) that is desired. In this article, we will discuss designing a lifting beam according to ASME BTH-1. Therefore, we are looking at a beam that is lifted from the centre and loaded at the ends. This type of lift subjects the beam to flexure (bending) and shear, with bending stress and lateral torsional buckling being the primary modes of failure.
Classification
After establishing the style and capacity required of the lifting beam, the Design Category and Service Class must be confirmed, according to ASME BTH-1. The design category and service class establish the requirements for safety factor for strength and fatigue that will be used in the remainder of the design calculations for the lifting device. The design category and service class are discussed in more detail in the article: Introduction to Below the Hook Design
Structural Design
ASME BTH-1 specifies design calculations for different types of loading of a lifting device including tension, compression, flexure, shear and combined loading of beams. For lifting beams that are subject to flexure, ASME BTH-1 has calculations that define the maximum allowable bending stress based on beam geometry, material properties, and lateral bracing spacing (the distance between braces that resist lateral displacement of the compression flange or twisting of the beam cross-section). Typically lifting beams do not have bracing to resist either of these movements (a single symmetric cantilever beam with no external bracing to stabilize the beam), therefore the lateral bracing spacing is defined as the distance between the outermost lift points of the lifting beam.
Lifting Beam Design Calculations Using
Based on the BTH-1 calculations, if the beam length is short enough the beam yield strength limits the lift capacity of the beam, but if the beam length is over the lateral bracing spacing requirement (per calculations in BTH-1), the buckling strength of the structural member defines the allowable lift capacity of the beam, resulting in a reduced maximum allowable bending stress.
Depending on the features of the lifting beam, additional calculations for connections (bolts, pins and welds) are specified to determine suitable sizing and spacing of each.
Calculations for fatigue are also specified in the standard, dependent on the service class of the bar and bar geometry.
The following chart shows a comparison of a W-Beam lifting beam with lifting capacity based on simple bending stress calculations vs calculations that account for buckling per ASME BTH-1. It shows how there is a reduction in lifting capacity in longer beams due to the instability from lateral torsional buckling.
Allowable bending stress vs beam length per BTH-1 calculations (Orange Plot)
According to BTH-1 calculations, the short beam can be loaded to full yield strength (accounting for safety factors), but as the beam length (or lifting spread) increases, the stress reaches an inflection point where the allowable bending stress starts to drop off. It is at this point where the beam buckling takes over as the limiting factor for load capacity. There are two other inflection points in the curve, one at a beam length of about 180 and another at a beam length of 255. These points are where calculations switch according to BTH-1, due to the beam length (There are several different calculations for allowable stress which are dependent on the beam properties and beam length, with different calculations for different lengths of beams).
Lift capacity vs beam length per BTH-1 calculations (Blue Plot)

The lift capacity of the beam is calculated based on the allowable stress (Orange Plot) and beam length. The calculation includes the weight of the beam.
Lift capacity vs beam length based only on bar yield strength (Gray Plot)
The allowable bending stress is a product of calculations from BTH-1, and the lift capacity is calculated from the allowable bending stress. The bending calculations include bar weight, but do not account for shear stresses. As well, as a comparison, the gray line represents the calculated beam lift capacity based on only bending strength, without accounting for buckling effects.
As can be see from the allowable stress plot, the short beam is permitted to achieve full bending stress (with safety factors), but at a certain point, the allowable bending stress is reduced, to account for the buckling strength of the beam.
Hey, you made it to the end! Congratulations! I hope this article makes you wonder if the engineers you are currently using are just tossing their lifting beam designs into solidworks and applying loads rather than doing the full calculations required by the code. If you are ready to skip the stress of designing your own lifting equipment take a second to check out our store: Basepoing Engineering Store Where you can find fully engineered stamped and certified drawings based on years of experience designing and building spreader bars.